Extra Questions for Class 9 Science Chapter 8 Motion
Extra questions for Class 9 Science Chapter 8 Motion with answers is given below. Our subject expert prepared these solutions as per the latest NCERT textbook. These questions will be helpful to revise the all topics and concepts. CBSE Class 9 extra questions are the most simple and conceptual questions that are prepared by subject experts for the students to study well for the final exams. By solving these extra questions, students can be very efficient in their exam preparations.
Motion Class 9 Science Extra Questions and Answers
Very Short Answer Questions
1: The phenomenon of motion was placed on a sound scientific footing by two scientists. Write their names.
Answer: Galileo Galilei and Isaac Newton.
2: Are rest and motion absolute or relative terms?
Answer: They are relative terms.
3: Suppose a ball is thrown vertically upwards from a position P above the ground. It rises to the highest point Q and returns to the same point P. What is the net displacement and distance travelled by the ball?
Answer: Displacement is zero. Distance is twice the distance between position P and Q.
4: Which speed is greater: 30 m/s or 30 km/h?
Answer: 30 m/s
5: What do you mean by 2 m/s2?
Answer: The velocity of the body increases by 2 m/s after every second.
6: Can uniform linear motion be accelerated?
Answer: No
7: Define one radian.
Answer: It is the angle which is subtended at the centre by an arc having a length equal to the radius of the circle.
8: What is the relation between linear velocity and angular velocity
Answer: Linear velocity = Angular velocity x Radius of circular path.
Short Answer Type Questions
1: Give an example of a body which may appear to be moving for one person and stationary for the other.
Answer: The passengers in a moving bus observe that the trees, buildings as well as the people on the roadside appear to be moving backwards. Similarly, a person standing on the roadside observes that the bus (along with its passengers) is moving in forward direction. But, at the same time, each passenger in a moving bus or train observes, his fellow passengers sitting and not moving. Thus, we can tell that motion is relative.
2: How can we describe the location of an object?
Answer: To describe the position of an object we need to specify a reference point called the origin. For example, suppose that a library in a city is 2 km north of the railway station. We have specified the position of the library with respect to the railway station i.e., in this case, the railway station acts as the reference point.
3: What do you mean by average speed? What are its units?
Answer: Average speed is defined as the average distance travelled per unit time and is obtained by dividing the total distance travelled by the total time taken. The unit of average speed is the same as that of the speed, that is, ms-1.
4: What is the difference between uniform velocity and non-uniform velocity?
Answer: Uniform velocity: An object with uniform velocity covers equal distances in equal intervals of time in a specified direction, e.g., an object moving with speed of 40km h-1 towards west has uniform velocity.
Non-uniform velocity: When an object covers unequal distances in equal intervals of time in a specified direction, or if the direction of motion changes, it is said to be moving with a non-uniform or variable velocity, e.g., revolving fan at a constant speed has variable velocity.
5: Differentiate between distance and displacement.
Answer:
| Distance | Displacement |
| It is the length of the actual path covered by an object, irrespective of its direction of motion. | Displacement is the shortest distance between the initial and final positions of an object in a given direction. |
| Distance is a scalar quantity | Displacement is a vector quantity. Displacement may be positive negative or zero. |
| Distance between two given points may be same or different for different path chosen. | Displacement between two given points is always the same. |
| Distance covered can never be negative. It is always positive or zero. | Displacement between two given points is always the same. |
6: What are the uses of a distance-time graph?
Answer: The various uses of a distance-time graph are as follows:
(a) It tells us about the position of the body at any instant of time.
(b) From the graph, we can see the distance covered by the body during a particular interval of time.
(c) It also gives us information about the velocity of the body at any instant of time.
7: A cyclist cycles for t second at a speed of 3 m/s and then for the same time at a speed of 5 m/s along a straight road due north. What is the average speed of the cyclist?
Answer: You may compute arithmetic mean to compute average speed.
Alternately,
Total Time taken = 2t seconds
Total Distance covered = 3t + 5t = 8t m

8: When will you say a body is in (i) uniform acceleration? (ii) nonuniform acceleration?
Answer: (i) A body is said to be in uniform acceleration if it travels in a straight line and its velocity increases or decreases by equal amounts in equal intervals of time.
(ii) A body is said to be in nonuniform acceleration if the rate of change of its velocity is not constant in equal intervals of time.
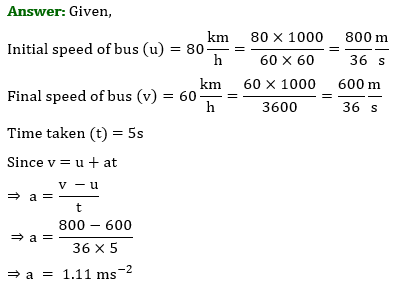
9: A bus decreases its speed from 80 km/h to 60 km/h in 5 s. Find the acceleration of the bus.
10: A train starting from a railway station and moving with uniform acceleration attains a speed 40 km/h in 10 minutes. Find its acceleration.

11: A bus starting from rest moves with a uniform acceleration of 0.1 ms–2 for 2 minutes.
Find (a) the speed acquired, (b) the distance travelled.
Answer: Given,
Initial speed (u) = 0 m/s
Acceleration of bus (a) = 0.1 ms–2
Time taken (t) = 2 min = 2 × 60 = 120 s
Final velocity = ?
Distance Covered (S) = ?
∴ v = u + at
= 0 + 0.1 × 120
= 12 m/s
∴ S = ut + ½at2
= 0 + (0.1 × 1202 )/2
= 0.1 × 14400 /2
= 720m
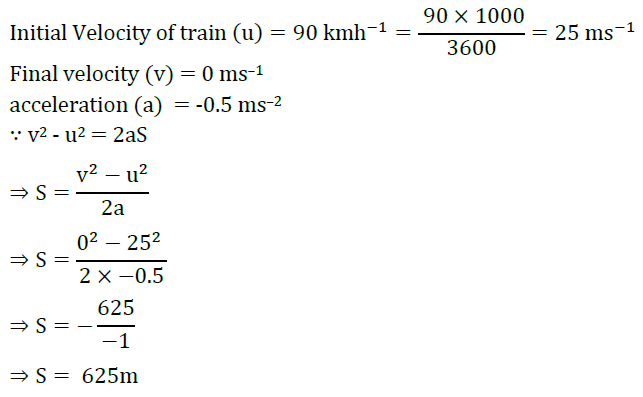
12: A train is travelling at a speed of 90 kmh–1. Brakes are applied so as to produce a uniform acceleration of – 0.5 ms–2. Find how far the train will go before it is brought to rest.
Answer: Given,
13: A trolley, while going down an inclined plane, has an acceleration of 2 cm s–2. What will be its velocity 3 s after the start?
Answer: Given,
Initial velocity (u) = 0 cm s–1.
acceleration (a) = 2 cm s–2.
time taken (t) = 3s
∴ v = u + at
⇒ v = 0 + 2 × 3
⇒ v = 6 cm s–1
14: A racing car has a uniform acceleration of 4 m s–2. What distance will it cover in 10 s after start?
Answer: Given,
Initial velocity of car (u) = 0 m s–1.
acceleration (a) = 4 m s–2.
time taken (t) = 10 s
Distance covered (S) = ?
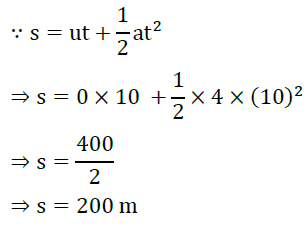
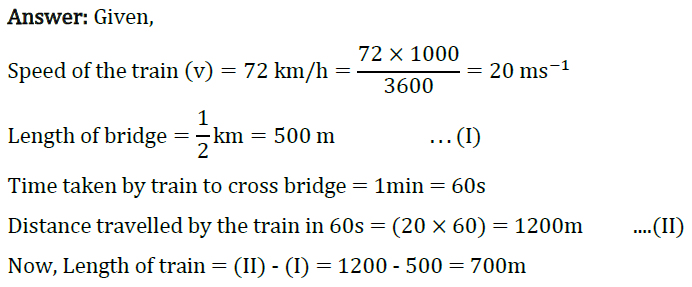
15: A train is running at a speed of 72 km/h. It crosses a bridge of length half kilometer in 1 minute. Calculate the length of the train.
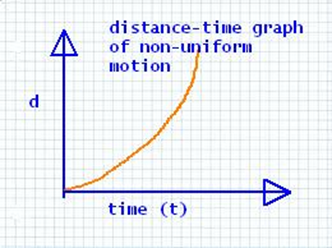
16: What is the nature of the distance-time graphs for uniform and non-uniform motion of an object?
Answer: (a) When an object moves with uniform (constant) speed, it covers equal distances in equal intervals of time. It will make a straight line on distance-time graph making an angle with time (x-axis) axis.
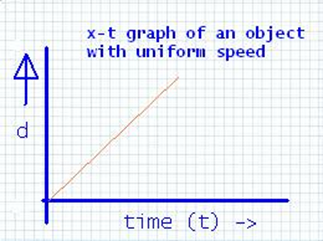
(b) For an object moving in a non-uniform motion will cover unequal distances in equal intervals of time. It will be a curve on a distance-time graph.
Long Answer Type Questions
1: With the help of a graph, derive the relation v = u + at.
Answer: Consider the velocity-time graph of an object that moves under uniform acceleration as shown in the figure (u≠0).
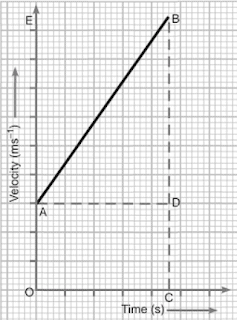
From this graph, we can see that initial velocity of the object (at point A) is u and then it increases to v (at point B) in time t. The velocity changes at uniform rate a. As shown in the figure, the lines BC and BE are drawn from point B on the time and the velocity axes respectively.
The initial velocity is represented by OA.
The final velocity is represented by BC.
The time interval t is represented by OC.
BD = BC – CD, represents the change in velocity in time interval t.
If we draw AD parallel to OC,
we observe that BC = BD + DC = BD + OA
Substituting, BC with v and OA with u, we get
v = BD + u
or, BD = v – u …(i)
Thus, from the given velocity-time graph, the acceleration of the object is given by Change in velocity
a = (Change in velocity)/(Time Taken)
= BD/AD
= BD/OC
Substituting OC with t, we get
a = BD/t
⇒ BD = at …(ii)
From equations (i) and (ii), we have
v-u = at
or, v =u + at